Rules for arithmetic operations on ordinary fractions. Operations with fractions

Already in elementary school, students are exposed to fractions. And then they appear in every topic. You cannot forget actions with these numbers. Therefore, you need to know all the information about ordinary and decimal fractions. These concepts are not complicated, the main thing is to understand everything in order.

Why are fractions needed?

The world around us consists of entire objects. Therefore, there is no need for shares. But everyday life constantly pushes people to work with parts of objects and things.

For example, chocolate consists of several pieces. Consider a situation where his tile is formed by twelve rectangles. If you divide it into two, you get 6 parts. It can easily be divided into three. But it will not be possible to give five people a whole number of chocolate slices.

By the way, these slices are already fractions. And their further division leads to the appearance of more complex numbers.

What is a "fraction"?

This is a number made up of parts of a unit. Outwardly, it looks like two numbers separated by a horizontal or slash. This feature is called fractional. The number written at the top (left) is called the numerator. What is at the bottom (right) is the denominator.

Essentially, the slash turns out to be a division sign. That is, the numerator can be called the dividend, and the denominator can be called the divisor.

What fractions are there?

In mathematics there are only two types: ordinary and decimal fractions. Schoolchildren become acquainted with the first ones in elementary school, calling them simply “fractions.” The latter will be learned in 5th grade. That's when these names appear.

Common fractions are all those that are written as two numbers separated by a line. For example, 4/7. A decimal is a number in which the fractional part has a positional notation and is separated from the whole number by a comma. For example, 4.7. Students need to clearly understand that the two examples given are completely different numbers.

Every simple fraction can be written as a decimal. This statement is almost always true in reverse. There are rules that allow you to write a decimal fraction as a common fraction.

What subtypes do these types of fractions have?

It is better to start in chronological order, as they are studied. Common fractions come first. Among them, 5 subspecies can be distinguished.

Correct. Its numerator is always less than its denominator.

Wrong. Its numerator is greater than or equal to its denominator.

Reducible/irreducible. It may turn out to be either right or wrong. Another important thing is whether the numerator and denominator have common factors. If there are, then it is necessary to divide both parts of the fraction by them, that is, reduce it.

Mixed. An integer is assigned to its usual regular (irregular) fractional part. Moreover, it is always on the left.

Composite. It is formed from two fractions divided by each other. That is, it contains three fractional lines at once.

Decimal fractions have only two subtypes:

finite, that is, one whose fractional part is limited (has an end);

infinite - a number whose digits after the decimal point do not end (they can be written endlessly).

How to convert a decimal fraction to a common fraction?

If this is a finite number, then an association is applied based on the rule - as I hear, so I write. That is, you need to read it correctly and write it down, but without a comma, but with a fractional bar.

As a hint about the required denominator, you need to remember that it is always one and several zeros. You need to write as many of the latter as there are digits in the fractional part of the number in question.

How to convert decimal fractions into ordinary fractions if their integer part is missing, that is, equal to zero? For example, 0.9 or 0.05. After applying the specified rule, it turns out that you need to write zero integers. But it is not indicated. All that remains is to write down the fractional parts. The first number will have a denominator of 10, the second will have a denominator of 100. That is, the given examples will have the following numbers as answers: 9/10, 5/100. Moreover, it turns out that the latter can be reduced by 5. Therefore, the result for it needs to be written as 1/20.

How can you convert a decimal fraction into an ordinary fraction if its integer part is different from zero? For example, 5.23 or 13.00108. In both examples, the whole part is read and its value is written. In the first case it is 5, in the second it is 13. Then you need to move on to the fractional part. The same operation is supposed to be carried out with them. The first number appears 23/100, the second - 108/100000. The second value needs to be reduced again. The answer gives the following mixed fractions: 5 23/100 and 13 27/25000.

How to convert an infinite decimal fraction to an ordinary fraction?

If it is non-periodic, then such an operation will not be possible. This fact is due to the fact that each decimal fraction is always converted to either a finite or a periodic fraction.

The only thing you can do with such a fraction is round it. But then the decimal will be approximately equal to that infinite. It can already be turned into an ordinary one. But the reverse process: converting to decimal will never give the initial value. That is, infinite non-periodic fractions are not converted into ordinary fractions. This needs to be remembered.

How to write an infinite periodic fraction as an ordinary fraction?

In these numbers, there are always one or more digits after the decimal point that are repeated. They are called a period. For example, 0.3(3). Here "3" is in the period. They are classified as rational because they can be converted into ordinary fractions.

Those who have encountered periodic fractions know that they can be pure or mixed. In the first case, the period starts immediately from the comma. In the second, the fractional part begins with some numbers, and then the repetition begins.

The rule by which you need to write an infinite decimal as a common fraction will be different for the two types of numbers indicated. It is quite easy to write pure periodic fractions as ordinary fractions. As with finite ones, they need to be converted: write down the period in the numerator, and the denominator will be the number 9, repeated as many times as the number of digits the period contains.

For example, 0,(5). The number does not have an integer part, so you need to immediately start with the fractional part. Write 5 as the numerator and 9 as the denominator. That is, the answer will be the fraction 5/9.

The rule on how to write an ordinary decimal periodic fraction that is mixed.

Look at the length of the period. That's how many 9s the denominator will have.

Write down the denominator: first nines, then zeros.

To determine the numerator, you need to write down the difference of two numbers. All numbers after the decimal point will be minified, along with the period. Deductible - it is without a period.

For example, 0.5(8) - write the periodic decimal fraction as a common fraction. The fractional part before the period contains one digit. So there will be one zero. There is also only one number in the period - 8. That is, there is only one nine. That is, you need to write 90 in the denominator.

To determine the numerator, you need to subtract 5 from 58. It turns out 53. For example, you would have to write the answer as 53/90.

How are fractions converted to decimals?

The simplest option is a number whose denominator is the number 10, 100, etc. Then the denominator is simply discarded, and a comma is placed between the fractional and integer parts.

There are situations when the denominator easily turns into 10, 100, etc. For example, the numbers 5, 20, 25. It is enough to multiply them by 2, 5 and 4, respectively. You just need to multiply not only the denominator, but also the numerator by the same number.

For all other cases, a simple rule is useful: divide the numerator by the denominator. In this case, you may get two possible answers: a finite or a periodic decimal fraction.

Operations with ordinary fractions

Addition and subtraction

Students become acquainted with them earlier than others. Moreover, at first the fractions have the same denominators, and then they have different ones. General rules can be reduced to this plan.

Find the least common multiple of the denominators.

Write additional factors for all ordinary fractions.

Multiply the numerators and denominators by the factors specified for them.

Add (subtract) the numerators of the fractions and leave the common denominator unchanged.

If the numerator of the minuend is less than the subtrahend, then we need to find out whether we have a mixed number or a proper fraction.

In the first case, you need to borrow one from the whole part. Add the denominator to the numerator of the fraction. And then do the subtraction.

In the second, it is necessary to apply the rule of subtracting a larger number from a smaller number. That is, from the module of the subtrahend, subtract the module of the minuend, and in response put a “-” sign.

Look carefully at the result of addition (subtraction). If you get an improper fraction, then you need to select the whole part. That is, divide the numerator by the denominator.

Multiplication and division

To perform them, fractions do not need to be reduced to a common denominator. This makes it easier to perform actions. But they still require you to follow the rules.

When multiplying fractions, you need to look at the numbers in the numerators and denominators. If any numerator and denominator have a common factor, then they can be reduced.

Multiply the numerators.

Multiply the denominators.

If the result is a reducible fraction, then it must be simplified again.

When dividing, you must first replace division with multiplication, and the divisor (second fraction) with the reciprocal fraction (swap the numerator and denominator).

Then proceed as with multiplication (starting from point 1).

In tasks where you need to multiply (divide) by a whole number, the latter should be written as an improper fraction. That is, with a denominator of 1. Then act as described above.



Operations with decimals

Addition and subtraction

Of course, you can always convert a decimal into a fraction. And act according to the plan already described. But sometimes it is more convenient to act without this translation. Then the rules for their addition and subtraction will be exactly the same.

Equalize the number of digits in the fractional part of the number, that is, after the decimal point. Add the missing number of zeros to it.

Write the fractions so that the comma is below the comma.

Add (subtract) like natural numbers.

Remove the comma.

Multiplication and division

It is important that you do not need to add zeros here. Fractions should be left as they are given in the example. And then go according to plan.

To multiply, you need to write the fractions one below the other, ignoring the commas.

Multiply like natural numbers.

Place a comma in the answer, counting from the right end of the answer as many digits as they are in the fractional parts of both factors.

To divide, you must first transform the divisor: make it a natural number. That is, multiply it by 10, 100, etc., depending on how many digits are in the fractional part of the divisor.

Multiply the dividend by the same number.

Divide a decimal fraction by a natural number.

Place a comma in your answer at the moment when the division of the whole part ends.



What if one example contains both types of fractions?

Yes, in mathematics there are often examples in which you need to perform operations on ordinary and decimal fractions. In such tasks there are two possible solutions. You need to objectively weigh the numbers and choose the optimal one.

First way: represent ordinary decimals

It is suitable if division or translation results in finite fractions. If at least one number gives a periodic part, then this technique is prohibited. Therefore, even if you don’t like working with ordinary fractions, you will have to count them.

Second way: write decimal fractions as ordinary

This technique turns out to be convenient if the part after the decimal point contains 1-2 digits. If there are more of them, you may end up with a very large common fraction and decimal notation will make the task faster and easier to calculate. Therefore, you always need to soberly evaluate the task and choose the simplest solution method.

Fraction- a number that consists of an integer number of fractions of a unit and is represented in the form: a/b

Numerator of fraction (a)- the number located above the fraction line and showing the number of shares into which the unit was divided.

Fraction denominator (b)- a number located under the fraction line and showing how many parts the unit is divided into.

2. Reducing fractions to a common denominator

3. Arithmetic operations on ordinary fractions

3.1. Addition of ordinary fractions

3.2. Subtracting fractions

3.3. Multiplying common fractions

3.4. Dividing fractions

4. Reciprocal numbers

5. Decimals

6. Arithmetic operations on decimals

6.1. Adding Decimals

6.2. Subtracting Decimals

6.3. Multiplying Decimals

6.4. Decimal division

#1. The main property of a fraction

If the numerator and denominator of a fraction are multiplied or divided by the same number that is not equal to zero, you get a fraction equal to the given one.

3/7=3*3/7*3=9/21, that is, 3/7=9/21

a/b=a*m/b*m - this is what the main property of a fraction looks like.

In other words, we get a fraction equal to the given one by multiplying or dividing the numerator and denominator of the original fraction by the same natural number.

If ad=bc, then two fractions a/b =c /d are considered equal.

For example, the fractions 3/5 and 9/15 will be equal, since 3*15=5*9, that is, 45=45

Reducing a fraction is the process of replacing a fraction in which the new fraction is equal to the original one, but with a smaller numerator and denominator.

It is customary to reduce fractions based on the basic property of the fraction.

For example, 45/60=15/ ​ ​20 =9/12=3/4 ​ ​ ​ (the numerator and denominator are divided by the number 3, by 5 and by 15).

Irreducible fraction is a fraction of the form 3/4 ​ ​ , where the numerator and denominator are mutually prime numbers. The main purpose of reducing a fraction is to make the fraction irreducible.

2. Reducing fractions to a common denominator

To bring two fractions to a common denominator, you need to:

1) factor the denominator of each fraction into prime factors;

2) multiply the numerator and denominator of the first fraction by the missing ones

factors from the expansion of the second denominator;

3) multiply the numerator and denominator of the second fraction by the missing factors from the first expansion.

Examples: Reduce fractions to a common denominator.

Let's factor the denominators into simple factors: 18=3∙3∙2, 15=3∙5

Multiply the numerator and denominator of the fraction by the missing factor 5 from the second expansion.

numerator and denominator of the fraction into the missing factors 3 and 2 from the first expansion.

= , 90 – common denominator of fractions.

3. Arithmetic operations on ordinary fractions

3.1. Addition of ordinary fractions

a) If the denominators are the same, the numerator of the first fraction is added to the numerator of the second fraction, leaving the denominator the same. As you can see in the example:

a/b+c/b=(a+c)/b ​ ​ ;

b) For different denominators, fractions are first reduced to a common denominator, and then the numerators are added according to rule a):

7/3+1/4=7*4/12+1*3/12=(28+3)/12=31/12

3.2. Subtracting fractions

a) If the denominators are the same, subtract the numerator of the second fraction from the numerator of the first fraction, leaving the denominator the same:

a/b-c/b=(a-c)/b ​ ​ ;

b) If the denominators of the fractions are different, then first the fractions are brought to a common denominator, and then the actions are repeated as in point a).

3.3. Multiplying common fractions

Multiplying fractions obeys the following rule:

a/b*c/d=a*c/b*d,

that is, they multiply the numerators and denominators separately.

For example:

3/5*4/8=3*4/5*8=12/40.

3.4. Dividing fractions

Fractions are divided in the following way:

a/b:c/d=a*d/b*c,

that is, the fraction a/b is multiplied by the inverse fraction of the given one, that is, multiplied by d/c.

Example: 7/2:1/8=7/2*8/1=56/2=28

4. Reciprocal numbers

If a*b=1, then the number b is reciprocal number for the number a.

Example: for the number 9 the reciprocal is 1/9 ​ ​ , since 9*1/9 = 1 , for the number 5 - the inverse number 1/5 ​ ​ , because 5* ​ 1/5 ​ ​ = 1 .

5. Decimals

Decimal is a proper fraction whose denominator is equal to 10, 1000, 10 000, …, 10^n 1 0 , 1 0 0 0 , 1 0 0 0 0 , . . . , 1 0 ​ n​ ​ .

For example: 6/10 =0,6; 44/1000=0,044 ​ .

Incorrect ones with a denominator are written in the same way 10^n or mixed numbers.

For example: 51/10= 5,1; 763/100=7,63

Any ordinary fraction with a denominator that is a divisor of a certain power of 10 is represented as a decimal fraction.

a changer, which is a divisor of a certain power of the number 10.

Example: 5 is a divisor of 100, so it is a fraction 1/5=1 *20/5*20=20/100=0,2 ​ 0 = 0 , 2 .

6. Arithmetic operations on decimals

6.1. Adding Decimals

To add two decimal fractions, you need to arrange them so that there are identical digits under each other and a comma under the comma, and then add the fractions like ordinary numbers.

6.2. Subtracting Decimals

It is performed in the same way as addition.

6.3. Multiplying Decimals

When multiplying decimal numbers, it is enough to multiply the given numbers, not paying attention to commas (like natural numbers), and in the resulting answer, a comma on the right separates as many digits as there are after the decimal point in both factors in total.

Let's multiply 2.7 by 1.3. We have 27\cdot 13=351 2 7 ⋅ 1 3 = 3 5 1 . We separate two digits on the right with a comma (the first and second numbers have one digit after the decimal point; 1+1=2 1 + 1 = 2 ). As a result we get 2.7\cdot 1.3=3.51 2 , 7 ⋅ 1 , 3 = 3 , 5 1 .

If the resulting result contains fewer digits than need to be separated by a comma, then the missing zeros are written in front, for example:

To multiply by 10, 100, 1000, you need to move the decimal point 1, 2, 3 digits to the right (if necessary, a certain number of zeros are assigned to the right).

For example: 1.47\cdot 10,000 = 14,700 1 , 4 7 ⋅ 1 0 0 0 0 = 1 4 7 0 0 .

6.4. Decimal division

Dividing a decimal fraction by a natural number is done in the same way as dividing a natural number by a natural number. The comma in the quotient is placed after the division of the whole part is completed.

If the integer part of the dividend is less than the divisor, then the answer is zero integers, for example:

Let's look at dividing a decimal by a decimal. Let's say we need to divide 2.576 by 1.12. First of all, let's multiply the dividend and divisor of the fraction by 100, that is, move the decimal point to the right in the dividend and divisor by as many digits as there are in the divisor after the decimal point (in this example, two). Then you need to divide the fraction 257.6 by the natural number 112, that is, the problem is reduced to the case already considered:

It happens that the final decimal fraction is not always obtained when dividing one number by another. The result is an infinite decimal fraction. In such cases, we move on to ordinary fractions.

For example, 2.8: 0.09= 28/10: 9/100= 28*100/10*9=2800/90=280/9= ​ 31 1/9 ​ ​ .

Organization: MBOU Bestuzhevskaya Secondary School

Locality: s. Bestuzhevo, Ustyansky district, Arkhangelsk region

Didactic material on the topic:

“Decimals. Operations with decimal fractions. Interest"

“Didactic material is a special type of visual teaching aid (mainly maps, tables, sets of cards with text, numbers or pictures, etc.), distributed to students for independent work in class or at home. Collections of tasks and exercises are also called didactic material."

• This didactic material was developed on the topic: “Decimal fractions. Operations with decimal fractions. Interest." is designed for 5th grade students of secondary schools and is intended for the formation and development of students' computing culture on this topic.

Target of this didactic material – students’ mastery of computational skills in working with decimals and percentages; development of cognitive activity and increase in educational motivation among fifth-graders; developing a culture of learning activity among students and increasing interest in mathematics.

Tasks:

1) To form and develop computational skills in working with decimals and percentages among fifth-graders when solving tasks of this didactic material;

2) To increase educational motivation and interest in studying mathematics among students through solving non-standard tasks of didactic material;

3) To develop cognitive activity and a culture of educational activity of students in various forms of working with this didactic material.

This didactic material is presented in the form of cards with various non-standard tasks. The first type of tasks is numerical crosswords. In these crossword puzzles, the answer can be a whole number or a finite decimal. Such crosswords are an alternative to examples from textbooks. When solving crossword puzzles, you need to perform an operation with decimal fractions, write the answer in the crossword puzzle, and keep in mind that each character is written in a separate cell. At the end of each crossword puzzle card there are instructions on how to fill in the answers. By solving such numerical crossword puzzles, students can control the correctness of their solutions (when working individually with a crossword puzzle) or control each other (when working in pairs or small groups). Crossword puzzles in the didactic material are presented on the following topics: “Writing decimals”, “Adding and subtracting decimals”, “Multiplying decimals by a natural number”, “Dividing decimals by a natural number”, “Multiplying decimals”, “Dividing a number” to a decimal."

The didactic material also contains tasks, the answer to which can be a word, phrase, saying or the name of a scientist. In such tasks, the student solves an example and receives an answer that corresponds to a specific letter. By solving all the examples in the task, you can get a term whose meaning is given below; a proverb or the name of a scientist who contributed to the development of mathematics. By solving such tasks, students will learn interesting facts from the history of mathematics, about various ancient counting devices, and about the history of interest. In the process of solving tasks, students can control the correctness of their decisions themselves or the teacher can control them. At the end of the task card there are instructions for filling out the answers. These tasks are educational in nature and are aimed at broadening the horizons of students. The didactic material contains tasks on the topics: “Adding and subtracting decimals”, “Multiplying decimals by a natural number”, “Multiplying and dividing decimals by a natural number”, “Multiplying decimals”, “Multiplying and dividing decimals”, “All operations with decimal fractions”, “Arithmetic average”, “Finding a number by its percentage”.

This didactic material contains tasks in which you need to insert missing numbers. This is a chain of calculations in which one number is given: the first, the last, or the number in the middle of the chain, and you need to arrange the remaining numbers, performing actions in one direction or the other. Chains of calculations are presented in different levels of complexity. This also includes tasks in which you need to insert missing numbers in a circle, performing various actions with the number in the center. Such tasks require control and verification by the teacher and are designed for oral calculation or small test work. These tasks are presented on the topics: “Adding and subtracting decimals”, “Multiplying and dividing decimals by natural numbers”, “Actions with decimals”, “Percents”.

The next type of tasks contained in the didactic material are tasks to determine the truth or falsity of a statement, which are also designed for oral solution or mathematical dictation. In such tasks, a statement is given or an example is solved and you need to determine whether it is true or false and put “I” or “L” in the circle next to the statement. When solving such tasks, students should be supervised by the teacher. The tasks are presented on the following topics: “Reading and writing decimal fractions”, “Multiplying a number by 0.1; 0.01; 0.001; …….”

The last type of tasks in this didactic material are tasks to find errors in examples or in solving equations. In such tasks you need to find and correct the proposed errors; each card with a self-control task indicates the number of mistakes made. The task is checked by the teacher. The tasks are presented on the topics: “Dividing decimal fractions by a natural number”, “Dividing a number by 0.1; 0.01; 0.001; …..”

When using non-standard tasks of this didactic material, students develop a computing culture, develop and practice computing skills on the topic: “Decimals. Operations with decimal fractions. Interest." Tasks of didactic material help to instill in students an interest in mathematics, increase their cognitive activity and motivation to learn. With the help of didactic material, fifth-graders develop the ability to independently comprehend and assimilate material on a given topic, and develop ingenuity. This didactic material can be used in lessons for students to work individually, work in pairs or small groups. For individual work, assignments are given to stronger students, weaker ones work in pairs or groups of 3-4 people. These tasks are assessed in different ways: self-assessment by students, mutual assessment when working in pairs or groups, assessment of work by the teacher. Didactic material assignments can be used for homework and self-preparation of students. Didactic material can be used at different stages of the lesson. At the stage of updating knowledge, chains of calculations and tasks are used to determine the truth and falsity of statements, and these tasks can also be used when conducting mathematical dictations. Number crosswords and word, phrase, or scientist name tasks can be used during the consolidation and application phases. This didactic material can be used to control and test students’ knowledge on the topic: “Decimal fractions. Operations with decimal fractions. Interest." When solving this type of task, students develop a culture of learning activity: if this is individual work, then the student independently determines the steps to solve and can control and evaluate himself, and can show ingenuity; if this is work in pairs or in a small group, then students distribute tasks among themselves, control each other, and conduct mutual assessment. Didactic material is aimed at self-control on the part of students, mutual control and training in the process of mastering educational material. When working with didactic material, the student solves a specific didactic problem using his knowledge and skills, while developing his intellectual, motivational, volitional and emotional spheres. From the experience of using this didactic material, I can say that students accept these tasks with a bang, and especially love to solve numerical crosswords.

When using this didactic material in the learning process, students form all groups of UUD (universal learning activities). UUD is a set of methods of action of a student (as well as associated learning skills), ensuring his ability to independently acquire new knowledge and skills, including the organization of this process. Formed and developed:

Personal UUD– use of acquired knowledge, motivation to learn, evaluation of one’s own educational activities.

Regulatory UUD- organization and planning of one’s educational activities, independent analysis of the conditions for achieving the goal, forecasting and anticipation of the result, control and correction of one’s activities.

Cognitive UUD - structuring knowledge, choosing the most effective ways to solve problems depending on specific conditions, proficiency in analysis and synthesis, searching and isolating the necessary information.

Communicative UUD - the ability to formulate thoughts, planning educational cooperation with the teacher and peers, managing the partner’s behavior - control, correction, evaluation of the partner’s actions, the ability to defend one’s point of view.

This didactic material was developed based on mathematics textbooks for grade 5: “Mathematics 5” by the team of authors Vilenkin N. Ya., Zhokhov V. I., Chesnokov A. S., Shvartsburd S. I., as well as “Mathematics 5” by the team authors Merzlyak A. G., Polonsky V. B., Yakir M. S. The tasks of the didactic material can be used by teachers in the process of teaching mathematics in grade 5 using textbooks by other authors. Also, didactic material will serve as a good assistant in students’ self-preparation. At the end of the didactic material, answers to the assignments are provided.

Bibliography:

1. Vilenkin N. Ya., Zhokhov V. I., Chesnokov A. S., Shvartsburd S. I. Mathematics 5th grade, 6th grade, textbook Moscow Mnemosyne, 2013.

2. Glazer G.I. History of mathematics at school. M.: Education, 1981.

3. Merzlyak A. G., Polonsky V. B., Yakir M. S. Mathematics 5, 6 grades. Moscow Ventana-Graf, 2013.

4. Merzlyak A. G., Polonsky V. B., Rabinovich E. M., Yakir M. S.. Didactic materials. Mathematics 5th grade, 6th grade. Moscow Ventana-Graf, 2015.

5. Rapatsevich E. S. The newest psychological and pedagogical dictionary. Modern school, 2010.

6. The fundamental core of the content of general education, edited by Kozlov V.V., Kondakov A.M.M.: Education 2011.

7. Chesnokov A. S., Neshkov K. I. Didactic materials in mathematics 5th grade, 6th grade. Moscow Classic Style, 2010.

8. Wikipedia. Free encyclopedia. https://ru.wikipedia.org/wiki/

§ 31. Problems and examples for all operations with decimal fractions.

Follow these steps:

767. Find the quotient of division:

772. Calculate:

Find X , If:

776. The unknown number was multiplied by the difference between the numbers 1 and 0.57 and the product was 3.44. Find the unknown number.

777. The sum of the unknown number and 0.9 was multiplied by the difference between 1 and 0.4 and the product was 2.412. Find the unknown number.

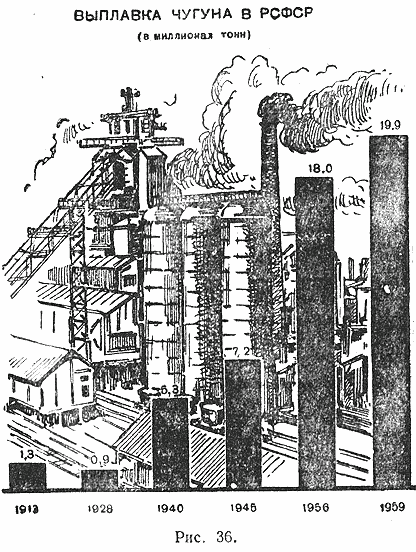
778. Using the data from the diagram about iron smelting in the RSFSR (Fig. 36), create a problem to solve which you need to apply the actions of addition, subtraction and division.

779. 1) The length of the Suez Canal is 165.8 km, the length of the Panama Canal is 84.7 km less than the Suez Canal, and the length of the White Sea-Baltic Canal is 145.9 km more than the length of the Panama Canal. What is the length of the White Sea-Baltic Canal?

2) The Moscow metro (by 1959) was built in 5 stages. The length of the first stage of the metro is 11.6 km, the second -14.9 km, the length of the third is 1.1 km less than the length of the second stage, the length of the fourth stage is 9.6 km more than the third stage, and the length of the fifth stage is 11.5 km less fourth. What was the length of the Moscow metro at the beginning of 1959?

780. 1) The greatest depth of the Atlantic Ocean is 8.5 km, the greatest depth of the Pacific Ocean is 2.3 km greater than the depth of the Atlantic Ocean, and the greatest depth of the Arctic Ocean is 2 times less than the greatest depth of the Pacific Ocean. What is the greatest depth of the Arctic Ocean?

2) The Moskvich car consumes 9 liters of gasoline per 100 km, the Pobeda car consumes 4.5 liters more than the Moskvich, and the Volga is 1.1 times more than the Pobeda. How much gasoline does a Volga car consume per 1 km of travel? (Round answer to the nearest 0.01 l.)

781. 1) The student went to his grandfather during the holidays. He traveled by rail for 8.5 hours, and from the station by horse for 1.5 hours. In total he traveled 440 km. At what speed did the student travel on the railroad if he rode horses at a speed of 10 km per hour?

2) The collective farmer had to be at a point located at a distance of 134.7 km from his home. He rode the bus for 2.4 hours at an average speed of 55 km per hour, and walked the rest of the way at a speed of 4.5 km per hour. How long did he walk?

782. 1) Over the summer, one gopher destroys about 0.12 centners of bread. In the spring, the pioneers exterminated 1,250 ground squirrels on 37.5 hectares. How much bread did the schoolchildren save for the collective farm? How much saved bread is there per 1 hectare?

2) The collective farm calculated that by destroying gophers on an area of ​​15 hectares of arable land, schoolchildren saved 3.6 tons of grain. How many gophers are destroyed on average per 1 hectare of land if one gopher destroys 0.012 tons of grain over the summer?

783. 1) When grinding wheat into flour, 0.1 of its weight is lost, and when baking, a bake equal to 0.4 of the weight of flour is obtained. How much baked bread will be produced from 2.5 tons of wheat?

2) The collective farm collected 560 tons of sunflower seeds. How much sunflower oil will be produced from the collected grains if the weight of the grain is 0.7 of the weight of sunflower seeds and the weight of the resulting oil is 0.25 of the weight of the grain?

784. 1) The yield of cream from milk is 0.16 of the weight of milk, and the yield of butter from cream is 0.25 of the weight of cream. How much milk (by weight) is required to produce 1 quintal of butter?

2) How many kilograms of porcini mushrooms must be collected to obtain 1 kg of dried mushrooms, if during preparation for drying 0.5 of the weight remains, and during drying 0.1 of the weight of the processed mushroom remains?

785. 1) The land allocated to the collective farm is used as follows: 55% of it is occupied by arable land, 35% by meadow, and the rest of the land in the amount of 330.2 hectares is allocated for the collective farm garden and for the estates of collective farmers. How much land is there on the collective farm?

2) The collective farm sowed 75% of the total sown area with grain crops, 20% with vegetables, and the remaining area with forage grasses. How much sown area did the collective farm have if it sowed 60 hectares with fodder grasses?

786. 1) How many quintals of seeds will be required to sow a field shaped like a rectangle 875 m long and 640 m wide, if 1.5 quintals of seeds are sown per 1 hectare?

2) How many quintals of seeds will be required to sow a field shaped like a rectangle if its perimeter is 1.6 km? The field width is 300 m. To sow 1 hectare, 1.5 quintals of seeds are required.

787. How many square plates with a side of 0.2 dm will fit in a rectangle measuring 0.4 dm x 10 dm?

788. The reading room has dimensions of 9.6 m x 5 m x 4.5 m. How many seats is the reading room designed for if 3 cubic meters are needed for each person? m of air?

789. 1) What area of ​​meadow will a tractor with a trailer of four mowers mow in 8 hours, if the working width of each mower is 1.56 m and the tractor speed is 4.5 km per hour? (Time for stops is not taken into account.) (Round the answer to the nearest 0.1 hectares.)

2) The working width of the tractor vegetable seeder is 2.8 m. What area can be sown with this seeder in 8 hours. work at a speed of 5 km per hour?

790. 1) Find the output of a three-furrow tractor plow in 10 hours. work, if the tractor speed is 5 km per hour, the grip of one body is 35 cm, and the waste of time was 0.1 of the total time spent. (Round the answer to the nearest 0.1 hectares.)

2) Find the output of a five-furrow tractor plow in 6 hours. work, if the tractor speed is 4.5 km per hour, the grip of one body is 30 cm, and the waste of time was 0.1 of the total time spent. (Round the answer to the nearest 0.1 hectares.)

791. The water consumption per 5 km of travel for a steam locomotive of a passenger train is 0.75 tons. The tender's water tank holds 16.5 tons of water. How many kilometers will the train have enough water to travel if the tank is filled to 0.9 of its capacity?

792. The siding can accommodate only 120 freight cars with an average car length of 7.6 m. How many four-axle passenger cars, each 19.2 m long, can fit on this track if 24 more freight cars are placed on this track?

793. To ensure the strength of the railway embankment, it is recommended to strengthen the slopes by sowing field grasses. For each square meter of embankment, 2.8 g of seeds are required, costing 0.25 rubles. for 1 kg. How much will it cost to sow 1.02 hectares of slopes if the cost of the work is 0.4 of the cost of the seeds? (Round the answer to the nearest 1 ruble.)

794. The brick factory delivered bricks to the railway station. 25 horses and 10 trucks worked to transport the bricks. Each horse carried 0.7 tons per trip and made 4 trips per day. Each vehicle transported 2.5 tons per trip and made 15 trips per day. The transportation lasted 4 days. How many bricks were delivered to the station if the average weight of one brick is 3.75 kg? (Round the answer to the nearest 1 thousand units.)

795. The flour stock was distributed among three bakeries: the first received 0.4 of the total stock, the second 0.4 of the remainder, and the third bakery received 1.6 tons less flour than the first. How much flour was distributed in total?

796. In the second year of the institute there are 176 students, in the third year there are 0.875 of this number, and in the first year there are one and a half times more than in the third year. The number of students in the first, second and third years was 0.75 of the total number of students of this institute. How many students were there at the institute?

___________

797. Find the arithmetic mean:

1) two numbers: 56.8 and 53.4; 705.3 and 707.5;

2) three numbers: 46.5; 37.8 and 36; 0.84; 0.69 and 0.81;

3) four numbers: 5.48; 1.36; 3.24 and 2.04.

798. 1) In the morning the temperature was 13.6°, at noon 25.5°, and in the evening 15.2°. Calculate the average temperature for this day.

2) What is the average temperature for the week, if during the week the thermometer showed: 21°; 20.3°; 22.2°; 23.5°; 21.1°; 22.1°; 20.8°?

799. 1) The school team weeded 4.2 hectares of beets on the first day, 3.9 hectares on the second day, and 4.5 hectares on the third. Determine the average output of the team per day.

2) To establish the standard time for manufacturing a new part, 3 turners were supplied. The first one produced the part in 3.2 minutes, the second in 3.8 minutes, and the third in 4.1 minutes. Calculate the time standard that was set for manufacturing the part.

800. 1) The arithmetic mean of two numbers is 36.4. One of these numbers is 36.8. Find something else.

2) The air temperature was measured three times a day: in the morning, at noon and in the evening. Find the air temperature in the morning if it was 28.4° at noon, 18.2° in the evening, and the average temperature of the day is 20.4°.

801. 1) The car traveled 98.5 km in the first two hours, and 138 km in the next three hours. How many kilometers did the average car travel per hour?

2) A test catch and weighing of yearling carp showed that out of 10 carp, 4 weighed 0.6 kg, 3 weighed 0.65 kg, 2 weighed 0.7 kg and 1 weighed 0.8 kg. What is the average weight of a yearling carp?

802. 1) For 2 liters of syrup costing 1.05 rubles. for 1 liter added 8 liters of water. How much does 1 liter of the resulting water with syrup cost?

2) The hostess bought a 0.5 liter can of canned borscht for 36 kopecks. and boiled with 1.5 liters of water. How much does a plate of borscht cost if its volume is 0.5 liters?

803. Laboratory work “Measuring the distance between two points”,

1st appointment. Measurement with a tape measure (measuring tape). The class is divided into units of three people each. Accessories: 5-6 poles and 8-10 tags.

Progress of work: 1) points A and B are marked and a straight line is drawn between them (see task 178); 2) lay the tape measure along the hung straight line and each time mark the end of the tape measure with a tag. 2nd appointment. Measurement, steps. The class is divided into units of three people each. Each student walks the distance from A to B, counting the number of his steps. By multiplying the average length of your step by the resulting number of steps, you find the distance from A to B.

3rd appointment. Measuring by eye. Each student extends his left hand with his thumb raised (Fig. 37) and points his thumb at the pole at point B (a tree in the picture) so that the left eye (point A), thumb and point B are on the same straight line. Without changing position, close your left eye and look at your thumb with your right. Measure the resulting displacement by eye and increase it by 10 times. This is the distance from A to B.

_________________

804. 1) According to the 1959 census, the population of the USSR was 208.8 million people, and the rural population was 9.2 million more than the urban population. How many urban and how many rural population were there in the USSR in 1959?

2) According to the 1913 census, the population of Russia was 159.2 million people, and the urban population was 103.0 million less than the rural population. What was the urban and rural population in Russia in 1913?

805. 1) The length of the wire is 24.5 m. This wire was cut into two parts so that the first part was 6.8 m longer than the second. How many meters long is each part?

2) The sum of two numbers is 100.05. One number is 97.06 more than the other. Find these numbers.

806. 1) There are 8656.2 tons of coal in three coal warehouses, in the second warehouse there are 247.3 tons of coal more than in the first, and in the third there are 50.8 tons more than in the second. How many tons of coal are in each warehouse?

2) The sum of three numbers is 446.73. The first number is less than the second by 73.17 and more than the third by 32.22. Find these numbers.

807. 1) The boat moved along the river at a speed of 14.5 km per hour, and against the current at a speed of 9.5 km per hour. What is the speed of the boat in still water and what is the speed of the river current?

2) The steamer traveled 85.6 km along the river in 4 hours, and 46.2 km against the current in 3 hours. What is the speed of the steamboat in still water and what is the speed of the river flow?

_________

808. 1) Two steamships delivered 3,500 tons of cargo, and one steamship delivered 1.5 times more cargo than the other. How much cargo did each ship carry?

2) The area of ​​two rooms is 37.2 square meters. m. The area of ​​one room is 2 times larger than the other. What is the area of ​​each room?

809. 1) From two settlements, the distance between which is 32.4 km, a motorcyclist and a cyclist simultaneously rode towards each other. How many kilometers will each of them travel before the meeting if the speed of the motorcyclist is 4 times the speed of the cyclist?

2) Find two numbers whose sum is 26.35, and the quotient of dividing one number by the other is 7.5.

810. 1) The plant sent three types of cargo with a total weight of 19.2 tons. The weight of the first type of cargo was three times the weight of the second type of cargo, and the weight of the third type of cargo was half as much as the weight of the first and second types of cargo combined. What is the weight of each type of cargo?

2) In three months, a team of miners extracted 52.5 thousand tons of iron ore. In March it was produced 1.3 times, in February 1.2 times more than in January. How much ore did the crew mine monthly?

811. 1) The Saratov-Moscow gas pipeline is 672 km longer than the Moscow Canal. Find the length of both structures if the length of the gas pipeline is 6.25 times greater than the length of the Moscow Canal.

2) The length of the Don River is 3.934 times greater than the length of the Moscow River. Find the length of each river if the length of the Don River is 1,467 km greater than the length of the Moscow River.

812. 1) The difference between two numbers is 5.2, and the quotient of one number divided by another is 5. Find these numbers.

2) The difference between two numbers is 0.96, and their quotient is 1.2. Find these numbers.

813. 1) One number is 0.3 less than the other and is 0.75 of it. Find these numbers.

2) One number is 3.9 more than another number. If the smaller number is doubled, it will be 0.5 of the larger one. Find these numbers.

814. 1) The collective farm sowed 2,600 hectares of land with wheat and rye. How many hectares of land were sown with wheat and how many with rye, if 0.8 of the area sown with wheat is equal to 0.5 of the area sown with rye?

2) The collection of two boys together amounts to 660 stamps. How many stamps does each boy's collection consist of if 0.5 of the first boy's stamps are equal to 0.6 of the second boy's collection?

815. Two students together had 5.4 rubles. After the first spent 0.75 of his money, and the second 0.8 of his money, they had the same amount of money left. How much money did each student have?

816. 1) Two steamships set out towards each other from two ports, the distance between which is 501.9 km. How long will it take them to meet if the speed of the first ship is 25.5 km per hour, and the speed of the second is 22.3 km per hour?

2) Two trains set off towards each other from two points, the distance between which is 382.2 km. How long will it take them to meet if the average speed of the first train was 52.8 km per hour, and the second one was 56.4 km per hour?

817. 1) Two cars left two cities at a distance of 462 km at the same time and met after 3.5 hours. Find the speed of each car if the speed of the first was 12 km per hour greater than the speed of the second car.

2) From two settlements, the distance between which is 63 km, a motorcyclist and a cyclist left at the same time towards each other and met after 1.2 hours. Find the speed of the motorcyclist if the cyclist was traveling at a speed 27.5 km per hour less than the speed of the motorcyclist.

818. The student noticed that a train consisting of a steam locomotive and 40 carriages passed by him for 35 seconds. Determine the speed of the train per hour if the length of the locomotive is 18.5 m and the length of the carriage is 6.2 m. (Give the answer accurate to 1 km per hour.)

819. 1) A cyclist left A for B at an average speed of 12.4 km per hour. After 3 hours 15 minutes. another cyclist rode out from B towards him at an average speed of 10.8 km per hour. After how many hours and at what distance from A will they meet if 0.32 the distance between A and B is 76 km?

2) From cities A and B, the distance between which is 164.7 km, a truck from city A and a car from city B drove towards each other. The speed of the truck is 36 km, and the speed of the car is 1.25 times higher. The passenger car left 1.2 hours later than the truck. After how much time and at what distance from city B will the passenger car meet the truck?

820. Two ships left the same port at the same time and are heading in the same direction. The first steamer travels 37.5 km every 1.5 hours, and the second steamer travels 45 km every 2 hours. How long will it take for the first ship to be 10 km from the second?

821. A pedestrian first left one point, and 1.5 hours after his exit a cyclist left in the same direction. At what distance from the point did the cyclist catch up with the pedestrian if the pedestrian was walking at a speed of 4.25 km per hour and the cyclist was traveling at a speed of 17 km per hour?

822. The train left Moscow for Leningrad at 6 o'clock. 10 min. morning and walked at an average speed of 50 km per hour. Later, a passenger plane took off from Moscow to Leningrad and arrived in Leningrad simultaneously with the arrival of the train. The average speed of the aircraft was 325 km per hour, and the distance between Moscow and Leningrad was 650 km. When did the plane take off from Moscow?

823. The steamer traveled along the river for 5 hours, and against the current for 3 hours and covered only 165 km. How many kilometers did he walk downstream and how many against the current, if the speed of the river flow is 2.5 km per hour?

824. The train has left A and must arrive at B at a certain time; having passed half the way and doing 0.8 km in 1 minute, the train was stopped for 0.25 hours; having further increased the speed by 100 m per 1 million, the train arrived at B on time. Find the distance between A and B.

825. From the collective farm to the city 23 km. A postman rode a bicycle from the city to the collective farm at a speed of 12.5 km per hour. 0.4 hours after this, the collective farm executive rode into the city on a horse at a speed equal to 0.6 of the postman’s speed. How long after his departure will the collective farmer meet the postman?

826. A car left city A for city B, 234 km away from A, at a speed of 32 km per hour. 1.75 hours after this, a second car left city B towards the first, the speed of which was 1.225 times greater than the speed of the first. How many hours after its departure will the second car meet the first?

827. 1) One typist can retype a manuscript in 1.6 hours, and another in 2.5 hours. How long will it take both typists to type this manuscript, working together? (Round the answer to the nearest 0.1 hour.)

2) The pool is filled with two pumps of different power. The first pump, working alone, can fill the pool in 3.2 hours, and the second in 4 hours. How long will it take to fill the pool if these pumps are running simultaneously? (Round answer to the nearest 0.1.)

828. 1) One team can complete an order in 8 days. The other one needs 0.5 time to complete this order. The third team can complete this order in 5 days. How many days will it take to complete the entire order if three teams work together? (Round answer to the nearest 0.1 day.)

2) The first worker can complete the order in 4 hours, the second 1.25 times faster, and the third in 5 hours. How many hours will it take to complete the order if three workers work together? (Round the answer to the nearest 0.1 hour.)

829. Two cars are working to clean the street. The first of them can clean the entire street in 40 minutes, the second requires 75% of the time of the first. Both machines started working at the same time. After working together for 0.25 hours, the second machine stopped working. How long after that did the first machine finish cleaning the street?

830. 1) One of the sides of the triangle is 2.25 cm, the second is 3.5 cm larger than the first, and the third is 1.25 cm smaller than the second. Find the perimeter of the triangle.

2) One of the sides of the triangle is 4.5 cm, the second is 1.4 cm less than the first, and the third side is equal to half the sum of the first two sides. What is the perimeter of the triangle?

831 . 1) The base of the triangle is 4.5 cm, and its height is 1.5 cm less. Find the area of ​​the triangle.

2) The height of the triangle is 4.25 cm, and its base is 3 times larger. Find the area of ​​the triangle. (Round answer to the nearest 0.1.)

832. Find the area of ​​the shaded figures (Fig. 38).

833. Which area is larger: a rectangle with sides 5 cm and 4 cm, a square with sides 4.5 cm, or a triangle whose base and height are each 6 cm?

834. The room is 8.5 m long, 5.6 m wide and 2.75 m high. The area of ​​windows, doors and stoves is 0.1 of the total wall area of ​​the room. How many pieces of wallpaper will be needed to cover this room if a piece of wallpaper is 7 m long and 0.75 m wide? (Round the answer to the nearest 1 piece.)

835. It is necessary to plaster and whitewash the outside of a one-story house, the dimensions of which are: length 12 m, width 8 m and height 4.5 m. The house has 7 windows measuring 0.75 m x 1.2 m each and 2 doors each measuring 0.75 m x 2.5 m. How much will the whole work cost if whitewashing and plastering is 1 sq. m. m costs 24 kopecks? (Round the answer to the nearest 1 ruble.)

836. Calculate the surface and volume of your room. Find the dimensions of the room by measuring.

837. The garden has the shape of a rectangle, the length of which is 32 m, the width is 10 m. 0.05 of the entire area of ​​​​the garden is sown with carrots, and the rest of the garden is planted with potatoes and onions, and an area 7 times larger than with onions is planted with potatoes. How much land is individually planted with potatoes, onions and carrots?

838. The vegetable garden has the shape of a rectangle, the length of which is 30 m and the width of 12 m. 0.65 of the entire area of ​​​​the vegetable garden is planted with potatoes, and the rest with carrots and beets, and 84 square meters are planted with beets. m more than carrots. How much land separately is there for potatoes, beets and carrots?

839. 1) The cube-shaped box was lined on all sides with plywood. How much plywood was used if the edge of the cube is 8.2 dm? (Round the answer to the nearest 0.1 sq. dm.)

2) How much paint will be needed to paint a cube with an edge of 28 cm, if per 1 sq. cm will 0.4 g of paint be used? (Answer, round to the nearest 0.1 kg.)

840. The length of a cast iron billet in the shape of a rectangular parallelepiped is 24.5 cm, width 4.2 cm and height 3.8 cm. How much do 200 cast iron billets weigh if 1 cubic. dm of cast iron weighs 7.8 kg? (Round answer to the nearest 1 kg.)

841. 1) The length of a box (with a lid) in the shape of a rectangular parallelepiped is 62.4 cm, width 40.5 cm, height 30 cm. How many square meters of boards were used to make the box, if waste boards amount to 0.2 of the surface area that should be covered with boards? (Round the answer to the nearest 0.1 sq. m.)

2) The bottom and side walls of the pit, which has the shape of a rectangular parallelepiped, must be covered with boards. The length of the pit is 72.5 m, width 4.6 m and height 2.2 m. How many square meters of boards were used for sheathing if the waste of boards constitutes 0.2 of the surface that should be sheathed with boards? (Round the answer to the nearest 1 sq.m.)

842. 1) The length of the basement, shaped like a rectangular parallelepiped, is 20.5 m, the width is 0.6 of its length, and the height is 3.2 m. The basement was filled with potatoes to 0.8 of its volume. How many tons of potatoes fit in the basement if 1 cubic meter of potatoes weighs 1.5 tons? (Round answer to the nearest 1 thousand.)

2) The length of the tank, shaped like a rectangular parallelepiped, is 2.5 m, the width is 0.4 of its length, and the height is 1.4 m. The tank is filled with kerosene to 0.6 of its volume. How many tons of kerosene are poured into the tank if the weight of kerosene in a volume is 1 cubic meter? m equals 0.9 t? (Round answer to the nearest 0.1 t.)

843. 1) How long can it take to renew the air in a room that is 8.5 m long, 6 m wide and 3.2 m high, if through a window in 1 second. passes 0.1 cubic meters. m of air?

2) Calculate the time required to refresh the air in your room.

844. The dimensions of the concrete block for building walls are as follows: 2.7 m x 1.4 m x 0.5 m. The void makes up 30% of the volume of the block. How many cubic meters of concrete will be required to make 100 such blocks?

845. Grader-elevator (machine for digging ditches) in 8 hours. The work makes a ditch 30 cm wide, 34 cm deep and 15 km long. How many diggers does such a machine replace if one digger can remove 0.8 cubic meters? m per hour? (Round the result.)

846. The bin in the shape of a rectangular parallelepiped is 12 m long and 8 m wide. In this bin, grain is poured to a height of 1.5 m. In order to find out how much all the grain weighs, they took a box 0.5 m long, 0.5 m wide and 0.4 m high, filled it with grain and weighed it. How much did the grain in the bin weigh if the grain in the box weighed 80 kg?

849. Construct a linear diagram of the growth of the urban population in the USSR, if in 1913 the urban population was 28.1 million people, in 1926 - 24.7 million, in 1939 - 56.1 million and in 1959 - 99, 8 million people.

850. 1) Make an estimate for the renovation of your classroom, if you need to whitewash the walls and ceiling, and paint the floor. Find out the data for drawing up an estimate (class size, cost of whitewashing 1 sq. m, cost of painting the floor 1 sq. m) from the school caretaker.

2) For planting in the garden, the school bought seedlings: 30 apple trees for 0.65 rubles. per piece, 50 cherries for 0.4 rubles. per piece, 40 gooseberry bushes for 0.2 rubles. and 100 raspberry bushes for 0.03 rubles. for a bush. Write an invoice for this purchase using the following example:

ANSWERS

Actions with fractions. In this article we will look at examples, everything in detail with explanations. We will consider ordinary fractions. We'll look at decimals later. I recommend watching the whole thing and studying it sequentially.

1. Sum of fractions, difference of fractions.

Rule: when adding fractions with equal denominators, the result is a fraction - the denominator of which remains the same, and its numerator will be equal to the sum of the numerators of the fractions.

Rule: when calculating the difference between fractions with the same denominators, we obtain a fraction - the denominator remains the same, and the numerator of the second is subtracted from the numerator of the first fraction.

Formal notation for the sum and difference of fractions with equal denominators:

Examples (1):

It is clear that when ordinary fractions are given, then everything is simple, but what if they are mixed? Nothing complicated...

Option 1– you can convert them into ordinary ones and then calculate them.

Option 2– you can “work” separately with the integer and fractional parts.

Examples (2):

More:

What if the difference of two mixed fractions is given and the numerator of the first fraction is less than the numerator of the second? You can also act in two ways.

Examples (3):

*Converted to ordinary fractions, calculated the difference, converted the resulting improper fraction to a mixed fraction.

*We broke it down into integer and fractional parts, got a three, then presented 3 as the sum of 2 and 1, with one represented as 11/11, then found the difference between 11/11 and 7/11 and calculated the result. The meaning of the above transformations is to take (select) a unit and present it in the form of a fraction with the denominator we need, then we can subtract another from this fraction.

Another example:

Conclusion: there is a universal approach - in order to calculate the sum (difference) of mixed fractions with equal denominators, they can always be converted to improper ones, then perform the necessary action. After this, if the result is an improper fraction, we convert it to a mixed fraction.

Above we looked at examples with fractions that have equal denominators. What if the denominators are different? In this case, the fractions are reduced to the same denominator and the specified action is performed. To change (transform) a fraction, the basic property of the fraction is used.

Let's look at simple examples:

In these examples, we immediately see how one of the fractions can be transformed to get equal denominators.

If we designate ways to reduce fractions to the same denominator, then we will call this one METHOD ONE.

That is, immediately when “evaluating” a fraction, you need to figure out whether this approach will work - we check whether the larger denominator is divisible by the smaller one. And if it is divisible, then we perform a transformation - we multiply the numerator and denominator so that the denominators of both fractions become equal.

Now look at these examples:

This approach is not applicable to them. There are also ways to reduce fractions to a common denominator; let’s consider them.

Method TWO.

We multiply the numerator and denominator of the first fraction by the denominator of the second, and the numerator and denominator of the second fraction by the denominator of the first:

*In fact, we reduce fractions to form when the denominators become equal. Next, we use the rule for adding fractions with equal denominators.

Example:

*This method can be called universal, and it always works. The only downside is that after the calculations you may end up with a fraction that will need to be further reduced.

Let's look at an example:

It can be seen that the numerator and denominator are divisible by 5:

Method THREE.

You need to find the least common multiple (LCM) of the denominators. This will be the common denominator. What kind of number is this? This is the smallest natural number that is divisible by each of the numbers.

Look, here are two numbers: 3 and 4, there are many numbers that are divisible by them - these are 12, 24, 36, ... The smallest of them is 12. Or 6 and 15, they are divisible by 30, 60, 90 .... The least is 30. The question is - how to determine this least common multiple?

There is a clear algorithm, but often this can be done immediately without calculations. For example, according to the above examples (3 and 4, 6 and 15) no algorithm is needed, we took large numbers (4 and 15), doubled them and saw that they are divisible by the second number, but pairs of numbers can be others, for example 51 and 119.

Algorithm. In order to determine the least common multiple of several numbers, you must:

- decompose each number into SIMPLE factors

— write down the decomposition of the BIGGER of them

- multiply it by the MISSING factors of other numbers

Let's look at examples:

50 and 60 => 50 = 2∙5∙5 60 = 2∙2∙3∙5

in the expansion of a larger number one five is missing

=> LCM(50,60) = 2∙2∙3∙5∙5 = 300

48 and 72 => 48 = 2∙2∙2∙2∙3 72 = 2∙2∙2∙3∙3

in the expansion of a larger number two and three are missing

=> LCM(48.72) = 2∙2∙2∙2∙3∙3 = 144

* The least common multiple of two prime numbers is their product

Question! Why is finding the least common multiple useful, since you can use the second method and simply reduce the resulting fraction? Yes, it is possible, but it is not always convenient. Look at the denominator for the numbers 48 and 72 if you simply multiply them 48∙72 = 3456. You will agree that it is more pleasant to work with smaller numbers.

Let's look at examples:

*51 = 3∙17 119 = 7∙17

the expansion of a larger number is missing a triple

=> NOC(51,119) = 3∙7∙17

Now let's use the first method:

*Look at the difference in the calculations, in the first case there are a minimum of them, but in the second you need to work separately on a piece of paper, and even the fraction you received needs to be reduced. Finding the LOC simplifies the work significantly.

More examples:

*In the second example it is clear that the smallest number that is divisible by 40 and 60 is 120.

RESULT! GENERAL COMPUTING ALGORITHM!

— we reduce fractions to ordinary ones if there is an integer part.

- we bring fractions to a common denominator (first we look at whether one denominator is divisible by another; if it is divisible, then we multiply the numerator and denominator of this other fraction; if it is not divisible, we act using the other methods indicated above).

- Having received fractions with equal denominators, we perform operations (addition, subtraction).

- if necessary, we reduce the result.

- if necessary, then select the whole part.

2. Product of fractions.

The rule is simple. When multiplying fractions, their numerators and denominators are multiplied:

Examples:

Task. 13 tons of vegetables were brought to the base. Potatoes make up ¾ of all imported vegetables. How many kilograms of potatoes were brought to the base?

Let's finish with the piece.

*I previously promised to give you a formal explanation of the main property of a fraction through a product, please:

3. Division of fractions.

Dividing fractions comes down to multiplying them. It is important to remember here that the fraction that is the divisor (the one that is divided by) is turned over and the action changes to multiplication:

This action can be written in the form of a so-called four-story fraction, because the division “:” itself can also be written as a fraction:

Examples:

That's all! Good luck to you!

Sincerely, Alexander Krutitskikh.